Potential energy and kinetic energy

Any object which moves towards the ground has potential energy which is transferred to kinetic energy. The type and amount of potential energy an object has depends upon the height it is held at and whether it has elasticity or electrical charge. The energy transfer which occurs during the movement of the object from height towards the ground involves the following three types of energy store.

• Elastic potential energy – any object with a shape which can be changed and reformed has elastic potential energy. These objects can be stretched and squashed but this requires energy. This energy is stored as elastic potential energy.
• Gravitational potential energy – when an object is raised up and held at height against the force of gravity, the energy is stored as gravitational potential energy. The greater the height, the more gravitational potential energy the object has. Gravitational potential energy is transferred to kinetic energy if the object falls towards the ground. An example of this is seen when a book is lifted and placed on a shelf. Work is done against gravity in lifting the book and energy has been transferred to the gravitational potential energy store of the book.
• Kinetic energy – every object that moves has kinetic energy, and this is sometimes referred to as movement energy. The amount of kinetic energy an object has depends upon its mass and the speed at which it is moving. The more mass the object has and the faster it is moving, the more kinetic energy it has.

Bouncing a tennis ball

One example of an activity which involves energy transfer between all three of these energy stores is the bouncing of a tennis ball on the surface of a table or the ground.

These energy transfers are shown and explained in the following diagram:

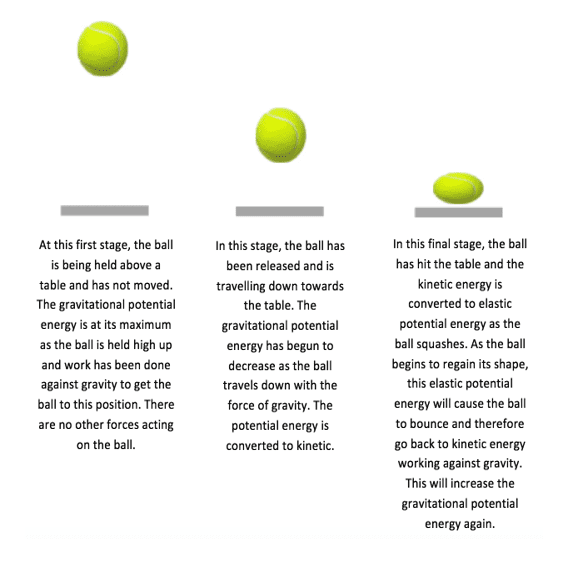
The gravitational potential energy is transferred to kinetic energy as the ball moves towards the table. The kinetic energy is then transferred to elastic potential energy as the ball is compressed. This elastic potential energy is then transferred to kinetic energy as the ball moves up again and bounces.

Pendulums

 A classic example of movement involving energy transfer between gravitational potential energy and kinetic energy stores, commonly used in exam questions, is the movement of a pendulum.

A pendulum is a simple device that can show the transfer of energy from gravity potential energy through to kinetic energy. When the pendulum is at its highest point of swing, it has zero kinetic energy but the gravitational potential energy is at its maximum. As the pendulum swings downwards, the gravitational potential energy is transferred to kinetic energy and the pendulum accelerates. As the pendulum is at the bottom of its swing, the kinetic energy is at its maximum and the gravitational potential energy is at its minimum. As the pendulum swings upwards, the kinetic energy is transferred to gravitational potential energy once again. Once the pendulum has reached the highest point of its swing, it once again has no kinetic energy but its gravitational potential energy is at a maximum. These energy transfers are shown in the diagram below:

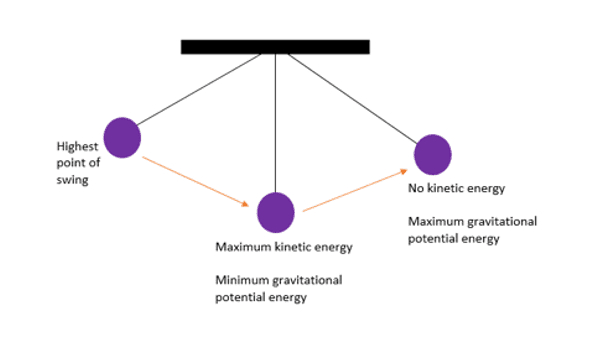
The amount of work done by the pendulum as it swings is equal to the amount of energy transferred.

The principle of energy states that energy can only be transferred and is never created nor destroyed.

This shows that the energy transferred from the gravitational potential energy store to the kinetic energy store should be equal. If this were the case, then the pendulum would continue to swing for an infinite amount of time.

However, over time the swing of the pendulum decreases in size and slows down. This is not because the energy is being destroyed but instead some of the kinetic energy is being transferred as thermal energy to the surroundings through the friction between the pendulum and the air particles.

The total amount of energy transferred to the kinetic energy stores and thermal energy stores is equal to the energy initially transferred from the gravitational energy stores.

As the pendulum slows down, the height it reaches becomes lower and the amount of gravitational potential energy decreases. As the amount of gravitational potential energy decreases, so does the amount of energy transferred to the kinetic energy store and the pendulum slows down.

Calculating gravitational potential energy (GPE)

The amount of gravitational potential energy an object has depends upon the object’s mass, the height from which it falls and the gravitational field strength.

Gravitational field strength is the strength of the gravitational field in which the object is found. The units for gravitational field strength are Newtons per kg (N/kg) and it is measured as the size of the force of gravity acting on an object in Newtons for every 1 kg of mass the object has.

The gravitational field strength is different for different places. For example, the gravitational field strength on Earth is 10 N/kg, whereas on the Moon it is 1.6N/kg.

A 10 kg object on Earth would have a gravitational force of 100 N, whereas on the Moon the same object would have a gravitational force of only 16 N. The relationship between gravitational field strength and mass can be used to determine the gravitational potential energy of the object at any specific height.

The equation used to calculate the gravitational potential energy is:

Gravitational potential energy is measured in joules (J)

Mass is measured in kilograms (kg)

Gravitational field strength is measured in Newtons per kilogram (N/kg)

Height is measure in metres (m)

You must know and be able to use this equation as it is not provided in the exam.

Example

A diver with a body mass of 65 kg stands on the edge of a diving board 30 metres above the water. The gravitational field strength on Earth is 10 N/kg.

Use this information to calculate how much gravitational potential energy the diver has.

Calculating kinetic energy (KE)

Kinetic energy is the energy that a moving object has. It can be defined as the amount of energy transferred or work needed to move an object at a specific speed.

The amount of kinetic energy needed depends upon the mass of the object and the speed at which it will be travelling.

The equation used to calculate kinetic energy is:

Kinetic energy is measured in joules (J)

Mass is measured in kilograms (kg)

Speed is measured in metres/second (m/s)

You must know and be able to use this equation as it will not be provided in the exam.

Example

Calculate the kinetic energy of a meteorite with a mass of 400 kg, moving towards the Earth at a speed of 2,000 m/s.